The spot VIX index finished last Friday at 11.28, a relatively low number, while the SKEW index was making a new high. The SKEW index is a good proxy for the cost of insurance and right now it appears to be expensive. A high reading of SKEW means investors are buying out of the money puts for protection.
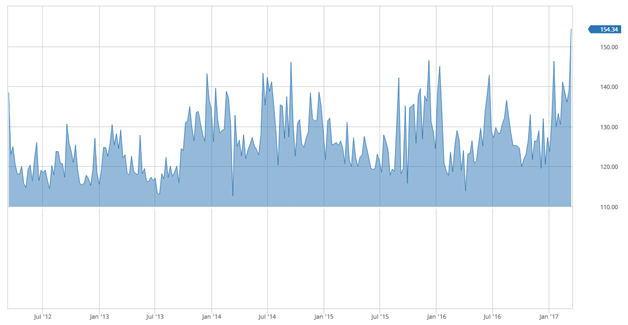
With the cost of insurance so high, is there a less expensive way for investors to hedge their portfolios?
One might think immediately of cross-asset hedging. However, if we use other underlying to hedge, we will then take on correlation (basis) risk and if not managed correctly, it can add risks to the portfolio instead of protecting it.
In this post we examine different hedging strategies using instruments on the same underlying. Our goal is to investigate the cost, risk/reward characteristics of each hedging strategy. Knowing the risk/reward profiles will allow us to design a cost-effective portfolio-protection scheme.
We will use Monte Carlo (MC) simulation to accomplish our goal [1]. The parameters and assumptions of the MC simulation are as follows:
| Parameter | Value |
| Initial stock price | 100 |
| Volatility | 20% |
| Risk-free rate | 0.02 |
| Drift | 0.07 |
| Days in simulation | 252 |
| Time step (day) | 1 |
| Number of paths | 10000 |
| Model | GBM [2] |
The hedging strategies we’re investigating are:
1-NO HEDGE: no hedging is performed. The asset is allowed to evolve freely in a risky world. This would correspond to the portfolio of a Buy and Hold investor.
2-PPUT: protective put. We buy an at the money (ATM) put in order to hedge the downside. This strategy is the most common type of portfolio insurance.
3-GAMMA: convexity hedge. We buy an ATM put, but we then dynamically hedge it. This means that we flatten out the delta at the end of every day.
The GAMMA hedging strategy is not used frequently in the industry. The rationale for introducing it here is that given a high price of a put option, we will try to partially recoup its cost by actively scalping gamma, while we still benefit from the positive convexity of the option. This means that in case of a market correction, the gamma will manufacture negative delta so that the hedging position can offset some of the loss in the equity portfolio.
We use 10000 paths in our MC simulation. At the end of 1 year, we calculate the returns (using a Reg-T account) and determine its mean and variance. We also calculate the Value at Risk at 95% confidence interval. The graph below shows the histogram of the returns for the NO HEDGE strategy,
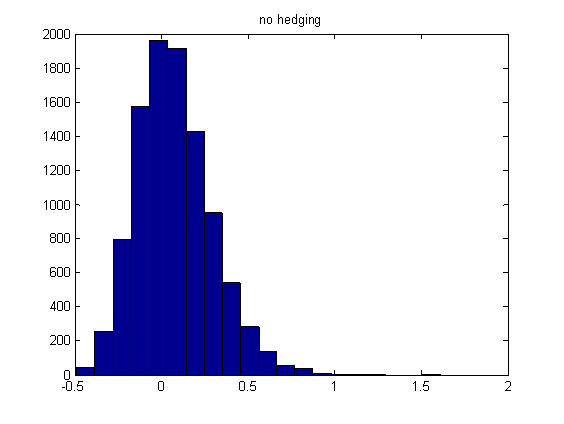
Table below presents the expected returns, standard deviations and Value at Risks for the hedging strategies.
| Strategies | Expected return | Standard Deviation | Value at Risk |
| NO HEDGE | 0.075 | 0.048 | 0.318 |
| PPUT | 0.052 | 0.024 | 0.118 |
| GAMMA | 0.066 | 0.029 | 0.248 |
As it is observed from the table, hedging with a protective put (PPUT) reduces the risks. Standard deviation and VaR are reduced from 0.048 and 0.318 to 0.024 to 0.118 respectively. However, the expected return is also reduced, from 0.075 to 0.052. This reduction is the cost of the insurance.
Interestingly, hedging using gamma convexity (GAMMA strategy) provides some reduction in risks (Standard deviation of 0.029, and VaR of 0.248), while not diminishing the returns greatly (expected return of 0.066).
In summary, GAMMA hedging is a strategy that is worth considering when designing a portfolio insurance scheme. It’s a good alternative to the often used protective (and expensive) put strategy.
Footnotes
[1] We note that the simulations were performed under idealistic assumptions, some are advantageous, and some are disadvantageous compared to a real life situation. However, results and the conclusion are consistent with our real world experience.
[2] GBM stands for Geometric Brownian Motion.

Great idea and great use of MC to demonstrate. While the delta hedging should be cheapish I worry about the cost of buying the options.
Thanks for your comment. Yes, equity index options are generally overpriced. So if you buy them, and delta hedge, overtime you still lose money (i.e. the strategy has a negative expectancy). The idea here is not to use this strategy as standalone, but as a hedge for a Buy and Hold portfolio. The hedge still costs money, but less than a protective put.
I would like your opinion/corrections on a simple strategy:
Sell all buy and hold positions, sell cash covered puts (200/300 SPX points OTM), and use the proceeds to buy calls to participate in any additional upside. If market drops a lot you will be put the SPX and again have long exposure but you saved 200/300 points of losses. At that point you can rinse and repeat. Yes, there is risk, but no hedge is perfect and this one appears cheap. I have not backtested this. On the rinse and repeat part you can buy VXX puts instead of SPX calls for a faster gains potential.
What do you think?
Thanks for asking. A sensible strategy is: sell OTM cash-secured put options on an index ETF (e.g. SPY). If you get exercised, then take the ETF and turn the position into a covered call, i.e. sell calls. Keep selling calls until the ETF is called away. At this point start selling puts again. Rinse and repeat. This strategy has a positive expectancy due to the rich premium of the put and the mean-reverting tendency of the index. So what you’re suggesting makes sense, just the put is too far OTM (hence they have very low delta). You might want to consider going closer to ATM, if not all of the position, then part of it (sort of diversification). As for the call, I prefer doing a call debit spread instead of a straight call, or a call debit spread on SVXY.
I forgot to mention that in a regular account there are tax implications on the sale of the buy and hold portfolio.
How would an OTM put and ratio backspread stack up against the 3 examples in your analysis?
Thanks
Gabor, good question. My very first thought was: since the OTM put has a high skew premium, in a long run the backspread will probably have a negative expectancy. But if we use it as a hedge, and not as a speculative vehicle, probably it will do its job and outperform other strategies. I will investigate this further and let you know of the outcome. Thanks.